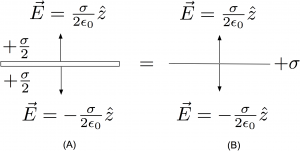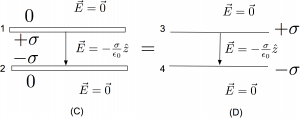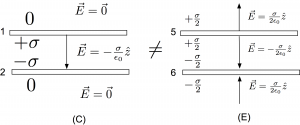Monthly Archives: November 2014

Knight in November video

Posted on by

I’ve recently released a new video for the song Knight in November off of the 2012 Agapanthus album Smug. I wrote the lyrics and music in the early 90s and recorded an ambient version of it circa 2002. This version was titled Night in November (not to be confused with the 1994 play by the same name) and also released on Smug. The original lyrics were about one particularly moody journey to Mount Hamilton’s Lick Observatory with a good friend. Driving up to Mount Hamilton was a frequent midnight pilgrimage in my youth while growing up in San Jose.

I recorded a heavier variant of the original song, now called Knight in November, in the summer of 2012. The lyrical verses sound like they just repeat the same phrase five times. But actually they form a set of (mostly) nonsensical homophones. Check out the video below to appreciate the effect. The tune can be downloaded for free from soundcloud (note the version on the album and soundcloud is a slightly different mix in the video).

Also below is the audio for the ambient piece, Night in November, from soundcloud. Hope you enjoy.

Knight In November from Thomas D. Gutierrez on Vimeo.

->

Thus spake Rankine: “U” for potential energy

Posted on by

Why is the symbol U often used to represent potential energy? This question came up recently in a faculty discussion.

Before you get too excited, this post won’t resolve the issue. However, the earliest use of the letter “U” for potential energy was in a paper from 1853 by William John Macquorn Rankine: “On the general law of the transformation of energy,” Proceedings of the Philosophical Society of Glasgow, vol. 3, no. 5, pages 276-280; reprinted in: (1) Philosophical Magazine, series 4, vol. 5, no. 30, pages 106-117 (February 1853). The wikipedia article on potential energy indicates that his article is the first reference to a modern sense of potential energy. Below is the original text (yellow highlighting mine). I think we will have to ask Bill Rankine why he chose the symbol “U”:

“Let U denote this potential energy.”
Thus spake Rankine.

screenshot_165

The field near a conductor

Posted on by

This post is directed primarily at physics students and instructors and stems from discussions with my colleague Prof. Matt Moelter at Cal Poly, SLO. In introductory electrostatics there is a standard result involving the electric field near conducting and non-conducting surfaces that confuses many students.

Near a non-conducting sheet of charge with charge density 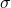\sigma, a straightforward application of gauss’s law gives the result

\vec{E}=\frac{\sigma}{2\epsilon_0}\hat{n}\ \ \ \ (1)&s=1

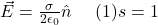
While, near the surface of a conductor with charge density 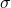\sigma, again, an application of gauss’s law gives the result

\vec{E}=\frac{\sigma}{\epsilon_0}\hat{n}\ \ \ \ (2)&s=1

The latter result comes about because the electric field inside a conductor in electrostatic equilibrium is zero, killing off the flux contribution of the gaussian pillbox inside the conductor. In the case of the sheet of charge, this same side of the pillbox distinctly contributed to the flux. Both methods are applied locally to small patches of their respective systems.

Although the two equations are derived from the same methods, they mean different things — and their superficial resemblance within factors of two can cause conceptual problems.

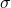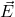In Equation (1) the relationship between \sigma and \vec{E} is causal. That is, the electric field is due directly from the source charge density in question. It does not represent the field due to all sources in the problem, only the lone contribution from that local \sigma.

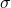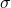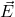In Equation (2) the relationship between \sigma and \vec{E} is not a simple causal one, rather it expresses self-consistentancy, discussed more below. Here the electric field represents the net field outside of the conductor near the charge density in question. In other words, it automatically includes both the contribution from the local patch itself and the contributions from all other sources. It is has already added up all the contributions from all other sources in the space around it (this could, in some cases, include sources you weren’t aware of!).

How did this happen? First, in contrast to the sheet of charge where the charges are fixed in space, the charges in a conductor are mobile. They aren’t allowed to move while doing the “statics” part of electrostatics, but they are allowed to move in some transient sense to quickly facilitate a steady state. In steady state, the charges have all moved to the surfaces and we can speak of an electrostatic surface distribution on the conductor. This charge mobility always arranges the surface distributions to ensure 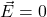\vec{E}=0 inside the conductor in electrostatic equilibrium. This is easy enough to implement mathematically, but gives rise to the subtle state of affairs encountered above. The 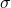\sigma on the conductor is responding to the electric fields generated by the presence of other charges in the system, but those other charges in the system are, in turn, responding to the local \sigma in question. Equation (2) then represents a statement of self-consistency, and it breaks the cycle using the power of gauss’s law. As a side note, the electric displacement vector, \vec{D}, plays a similar role of breaking the endless self-consistency cycle of polarization and electric fields in symmetric dielectric systems.

Let’s look at some examples.

Example 1:
Consider a large conducting plate versus large non-conducting sheet of charge. Each surface is of area A. The conductor has total charge Q, as does the non-conducting sheet. Find the electric field of each system. The result will be that the fields are the same for the conductor and non-conductor, but how can this be reconciled with Equation (1) and (2) which, at a glance, seem to give very different answers? See the figure below:

Conductor_1

For the non-conducting sheet, as shown in Figure (B) above, the electric field due to the source charge is given by Equation (1)

\vec{E}_{nc}=\frac{\sigma_{nc}}{2\epsilon_0}\hat{n}&s=1

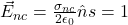
where

\sigma_{nc}\equiv\sigma=Q/A&s=1

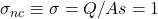
(“nc” for non-conducting) and 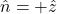\hat{n}=+\hat{z} above the positive surface and 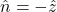\hat{n}=-\hat{z} below it.

Now, in the case of the conductor, shown in Figure (A), Equation (2) tells us the net value of the field outside the conductor. This net value is expressed, remarkably, only in terms of the local charge density; but remember, for a conductor, the local charge density contains information about the entire set of sources in the space. At a glance, it seems the electric field might be twice the value of the non-conducting sheet. But no! This is because the charge density will be different than the non-conducting case. For the conductor, the charge responds to the presence of the other charges and spreads out uniformly over both the top and bottom surface; this ensures \vec{E}=0 inside the conductor. In this context, it is worth point out that there are no infinitely thin conductors. Infinitely thin sheets of charge are fine, but not conductors. There are always two faces to a thin conducting surface and the surface charge density must be (at least tacitly) specified on each. Even if a problem uses language that implies the conducting surface is infinitely thin, it can’t be.

For example, the following Figure for an “infinitely thin conducting surface with charge density 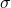\sigma“, which then applies Equation (2) to the setup to determine the field, makes no sense:

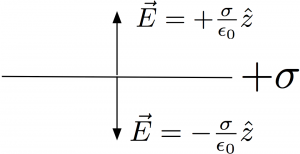
nonsenseconductor copy

This application of Equation (2) cannot be reconciled with Equation (1). We can’t have it both ways. An “infinitely thin conductor” isn’t a conductor at all and should reduce to Equation (1). To be a conductor, even a thin one, there needs to be (at least implicitly) two surfaces and a material medium we call “the conductor” that is independent of the charge.

Back to the example.

Conductor_1

If the charge Q is spread out uniformly over both sides of the conductor in Figure (A), the charge density for the conductor is then

\sigma_c=Q/2A=\sigma_{nc}/2=\sigma/2&s=1

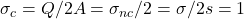
(“c” for conducting). The factor of 2 comes in because each face has area A and the charge spreads evenly across both. Equation (2) now tells us what the field outside the conductor is. This isn’t just for the one face, but includes the net contributions from all sources

\vec{E}_{c}=\frac{\sigma_c}{\epsilon_0}\hat{n}=\frac{\sigma_{nc}}{2\epsilon_0}\hat{n}=\vec{E}_{nc}&s=1.

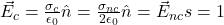That is, the net field is the same for each case,

\vec{E}_{c}=\vec{E}_{nc}&s=1.

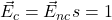Even though Equations (1) and (2) might seem superficially inconsistent with each other for this situation, they give the same answer, although for different reasons. Equation (1) gives the electric field that results directly from \sigma alone. Equation (2) gives a self consistent net field outside the conductor, which uses information contained in the local charge density. The key is understanding that the surface charge density used for the sheet of charge and the conductor are different in each case. In the case of a charged sheet, we have the freedom to declare a surface with a fixed, unchanging charge density. With a conductor, we have less, if any, control over what the charges do once we place them on the surfaces.

It is worth noting that each individual surface of charge on the conductor has a causal contribution to the field still given by Equation (1), but only once the surface densities have been determined — with one important footnote. The net field in each region can be determined by adding up all the (shown) individual contributions in superposition only if the charges shown are the only charges in the problem and were allowed to relax into this equilibrium state due to the charges explicitly shown. This last point will be illustrated in an example at the end of this post. It turns out that you can’t just declare arbitrary charge distributions on conductors and expect those same charges you placed to be solely responsible for it. There may be “hidden sources” if you insist on keeping your favorite arbitrary distribution on a conductor. If you do, you must also account for those contributions if you want to determine the net field by superposition. However, all is not lost: amazingly, Equation (2) still accounts for those hidden sources for the net field! With Equation (2) you don’t need to know the individual fields from all sources in order to determine the net field. The local charge density on the conductor already includes this information!

Example 2:
Compare the field between a parallel plate capacitor with thin conducting sheets each having charge 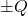\pm Q and area A with the field between two non-conducting sheets of charge with charge 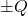\pm Q and area A. This situation is a standard textbook problem and forms the template for virtually all introductory capacitor systems. The result is that the field between the conducting plates are the same as the field between the non-conducting charge sheets, as shown in the figure below. But how can this be reconciled with Equations (1) and (2)? We use a treatment similar to those in Example 1.

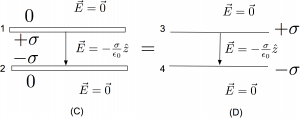
Plates

Between the two non-conducting sheets, as shown in Figure (D), the top positive sheet has a field given by Equation (1), pointing down (call this the 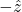-\hat{z} direction) . The bottom negative sheet also has a field given by Equation (1) and it also points down. The charge density on the positive surface is given by 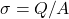\sigma=Q/A. We superimpose the two fields to get the net result

\vec{E}=\vec{E}_{1}+\vec{E}_2=\frac{+\sigma}{2\epsilon_0}(-\hat{z})+\frac{-\sigma}{2\epsilon_0}(+\hat{z})+=-\frac{\sigma}{\epsilon_0}(\hat{z})&s=1.

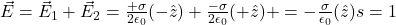Above the top positive non-conducting sheet the field points up due to the top non-conducting sheet and down from the negative non-conducting sheet. Using Equation (1) they have equal magnitude, thus the fields cancel in this region after superposition. The fields cancel in a similar fasshion below the bottom non-conducting sheet.

Unfortunately, the setup for the conductor, shown in Figure (C), is framed in an apparently ambiguous way. However, this kind of language is typical in textbooks. Where is this charge residing exactly? If this is not interpreted carefully, it can lead to inconsistencies like those of the “infinite thin conductor” above. The first thing to appreciate is that, unlike the nailed down charge on the non-conducting sheets, the charge densities on the parallel conducting plates are necessarily the result of responding to each other. The term “capacitor” also implies that we start with neutral conductors and do work bringing charge from one, leaving the other with an equal but opposite charge deficit. Next, we recognize even thin conducting sheets have two sides. That is, the top sheet has a top and bottom and the bottom conducting sheet also has a top and bottom. If the conducting plates have equal and opposite charges, and those charges are responding to each other. They will be attracted to each other and thus reside on the faces that are pointed at each other. The outer faces will contain no charge at all. That is, the 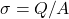\sigma=Q/A from the top plate is on that plate’s bottom surface with none on the top surface. Notice, unlike Example 1, the conductor has the same charge density as its non-conducting counterpart. Similar for the bottom plate but with the signs reversed. A quick application of gauss’s law can also demonstrate the same conclusion.

With this in mind, we are left with a little puzzle. Since we know the charge densities, do we jump right to the answer using Equation (2)? Or do we now worry about the individual contributions of each plate using Equation (1) and superimpose them to get the net field? The choice is yours. The easiest path is to just use Equation (2) and write down the results in each region. 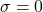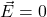Above and below all the plates, \sigma=0 so \vec{E}=0; again, Equation (2) has already done the superposition of the individual plates for us. In the middle, we can use either plate (but not both added…remember, this isn’t superposition!). If we used the top plate, we would get

\vec{E}=\frac{\sigma}{\epsilon_0}(-\hat{z})=-\frac{\sigma}{\epsilon_0}\hat{z}&s=1

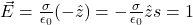
and if we used the bottom plate alone, we would get

\vec{E}=\frac{-\sigma}{\epsilon_0}\hat{z}=-\frac{\sigma}{\epsilon_0}\hat{z}&s=1.

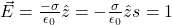They both give the same individual result, which is the same result as the non-conducting sheet case above where we added individual contributions.

If were were asked “what is the force of the top plate on the bottom plate?” we actually do need to know the field due to the charge on the single top plate alone and apply it to the charge on the second plate. In this case, we are not just interested in the total field due to all charges in the space as given by Equation (2). In this case, the field due to the single top plate would indeed be given by Equation (1), as would the field due to the single bottom plate. We could then go on to superimpose those fields in each region to obtain the same result. That is, once the charge distributions are established, we can substitute the sheets of non-conducting charge in place of the conducting plates and use those field configurations in future calculations of energy, force, etc.

However, not all charge distributions for the conductor are the same. A strange consequence of all this is that, despite the fact that Example 1 gave us one kind of conductor configuration that was equivalent to single non-conducting sheet, this same conductor can’t be just transported in and made into a capacitor as shown in the next figure:

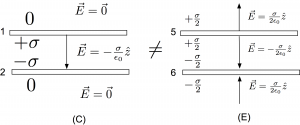
Conductor_3

On a conductor, we simply don’t have the freedom to invent a charge distribution, declare “this is a parallel plate capacitor,” and then assume the charges are consistent with that assertion. A charge configuration like Figure (E) isn’t a parallel plate capacitor in the usual parlance, although the capacitance of such a system could certainly be calculated. If we were to apply Equation (1) to each surface and superimpose them in each region, we might come to the conclusion that it had the same field as a parallel plate capacitor and conclude that Figure (E) was incorrect, particularly in the regions above and below the plates. However, Equation (2) tells us that the field in the region above the plates and below them cannot be zero despite what a quick application of Equation (1) might make us believe. What this tells us is that there must unseen sources in the space, off stage, that are facilitating the ongoing maintenance of this configuration. In other words, charges on conducting plates would not configure themselves in such a away unless there were other influences than the charges shown. If we just invent a charge distribution and impose it onto a conductor, we must be prepared to justify it via other sources, applied potentials, external fields, and so on.

So, even though plate (5) in Figure (E) was shown to be the same as a single non-conducting plate, we can’t just make substitutions like those in this figure. We can do this with sheets of charge, but not with other conductors. Yes, the configuration in Figure (E) is physically possible, it just isn’t the same as a parallel plate capacitor, even though each element analyzed in isolation makes it seem like it would be the same.

In short, Equations (1) and (2) are very different kinds of expressions. Equation (1) is a causal one that can be used in conjunction with the superposition principle: one is calculating a single electric field due to some source charge density. Equation (2) is more subtle and is a statement of self-consistency with the assumptions of a conductor in equilibrium. An application of Equation (2) for a conductor gives the net field due to all sources, not just the field do to the conducting patch with charge density sigma: it comes “pre-superimposed” for you.

Quick 4-dimensional visualization

Posted on by

How can you visualize a 4th spatial dimension? There has been much written and discussed on this topic; I won’t pretend that this post will compete with the vast resources available online. However, I do feel that I can contribute one small visualization trick for hypercubes that, for some reason, has not been emphasized very much elsewhere (although it is out there), which helped me get a foothold into the situation.

My first exposure as a kid to the topic of visualizing higher dimensions was given by Carl Sagan on the original Cosmos. In it, he introduces a hypercube called a tesseract:

While Cosmos is an inspirational introduction, it isn’t very complete. Still, there are many great resources on the web to help appreciate and understand the tesseract on many levels from rotations to inversions and beyond. They are part of a larger class of very cool objects known as polytopes. You are one google search away from vast resources on this topic. I won’t even bother compiling links.

What I hope to accomplish is to give you an intellectual foothold into the visualization, which will help considerably as you delve further into the topic.

Below is an image of a tesseract taken from the Wikipedia page on tesseracts
Schlegel_wireframe_8-cell

In what sense is this object a hypercube? Well, strictly speaking, this object is not a tesseract or hypercube. Technically, it is a two-dimensional projection (i.e. it is on this web page) of a three-dimensional shadow (the wire frame object if it were in 3D) of a 4D hypercube.

But how exactly can this object help us see into a 4th spatial dimension? Here is a visualization trick I’ve found most helpful for me:

Let’s start with something familiar. I can draw two parallelograms, one larger than the other, then connect the corresponding vertices. One’s mind will quickly interpret this as a cube as viewed from some angle, although it is just a two dimensional thing on a page. Your mind naturally views the (slightly) smaller parallelogram as being the same actual size as the larger one. It just looks smaller because we interpret it as farther away, thanks to perspective. Furthermore, all the angles, although drawn otherwise, are interpreted as right angles. The description makes it sound more complex than it is; it is just the representation of an ordinary cube viewed from some angle outside the page:

Cube1

In the drawing, the parallelograms are almost the same size, so it is easy to flip back and forth between which one is the “front” face and which is the “back” face, generating weird distortions if it is viewed “incorrectly.”

Now, I rotate the cube so we are looking directly down one face. Think of this drawing as looking down a crude wirefame corridor:
Cube2

However, on the page it is really just two nested squares with connected vertices. Still, one’s brain fills in the three dimensional details pretty naturally. Viewed this way, the smaller square is just further away and the angles are all right angles. If the smaller square were made smaller, even going to a point, you could imagine that the end of the corridor was just very far away.

The tesseract projection really is not really much different:

Schlegel_wireframe_8-cell

The visualization tool to remember is that the smaller cube only looks smaller because of perspective: the two cubes are actually the same size but the smaller cube only looks smaller because it is farther away. Further away in what direction? Into a 4th spatial dimension! When looking at the tesseract projection, think of it as looking “down” a kind of wireframe corridor directed such that the farthest point is actually at the mutual center of the cubes. This is the same sense that an ordinary long corridor drawn in two dimensions would have the far point (at infinity) located at the center of the squares. This mutual center is then interpreted as pointing in a direction not in ordinary three dimensional space; indeed, all six faces of the larger cube look “down” this corridor toward the other end. If you had such a hypercube in your living room, each of the six faces would act as a separate corridor directed towards the far point in a fourth spatial dimension. If your friend walked into the cube and continued down the corridor, they would not exit on the other side of the cube in your living room but rather would get smaller and smaller walking toward the center of the cube.

If you were the one doing the walking, it would be just like walking down a corridor into another room, albeit one that was entirely embedded — from all directions — within another one in three dimensions.

This is basically the idea behind Dr. Who’s tardis, as explained by the Doctor himself (although in his usual curt and opaque way):

You could think of the outer cube a crude 2 x 2 x 2 meter exterior to a tardis. The inner cube might be a 2 x 2 x 2 meter room inside the tardis (the same shape as the outside) 2 meters away into the 4th dimension. However, the tardis isn’t a mere hypercube. It has rooms inside that are bigger than the outside of the tardis. But to get them to fit inside the outer cube, you just put them farther away into the extra dimension. That is, you can visualize the inner cube as being a 100 x 100 x 100 meter room inside a 2 x 2 x 2 meter exterior box — except imagine you are 1000 meters away looking “down” the corridor of the 4th dimension, so the giant room looks small and thus fits fine into the exterior. This is exactly the point the Doctor is trying to make in the clip.

This idea was also a part of the plot of Stranger in a Strange Land by Heinlein. Valentine Michael Smith can make things vanish into a fourth dimension. The effect, as viewed by all observers in our own three dimensions, is to see the object get smaller and smaller from all angles until it vanishes. This is akin to walking down the corridor of the tesseract towards the center. The object appears to get smaller only because it is further away in this other direction outside of our usual three.

In my opinion, visualizing the tesseract as looking down a corridor into another spatial dimension with added perspective is the best first step in appreciating higher dimensional thinking. Here is a neat looking game that emphasizes the perspective approach and gives some practical practice with these ideas.

Update: Sean Carroll also just posted something on tesseracts on his blog Nov. 7.

Sexual harassment in NYC measuring mental illness?

Posted on by

An upsetting video (SFW) by Rob Bliss shows a woman being repeatedly verbally harassed as she walks the streets of New York City. The video is an edited sample of a 10 hour experiment. The actress, Shoshana B. Roberts, and Bliss were working on a project for Hollaback, an advocacy group trying to end street harassment. According to Bliss, who used a hidden camera and discreetly walked several paces in front of Roberts, the actress was harassed about 100 times during her 10 hour walk around the City (not all are shown in the video). In the video, one can clearly see Roberts simply walking and looking forward, minding her own business, not engaging or inviting conversation or interaction. Yet various men constantly vie for her attention, sometimes very aggressively, using a spectrum of nearly universally inappropriate strategies. This included many expressions like unsolicited neutral comments, catcalls, inappropriate remarks (usually about her looks), aggressive talking, shouting, following, and so on. The Washington Post has a good article summarizing the project and players. Here is the original video

A similar project was done on The Daily Show by comedian and correspondent Jessica Williams

I personally found the videos very disturbing and significant on many levels. They have helped me appreciate the issues women face while just walking from point A to B. Yes, as a man I have to navigate the occasional nuisance while walking along the street, but nothing like those shown in the videos. If these projects represent typical experiences for women, this represents a serious social problem. Even if it is atypical, a notion these videos do not support (the women in the videos seem “typical” — for example, no one is a recognizable popular celebrity whose presence might be especially socially disruptive), it is still upsetting. No one should need to experience interactions like that just walking around (including celebrities).

While emotionally impactful, it is important to realize the videos in no way represent a scientific experiment. There is no baseline measurement or control group. However, the video below might be a pretty decent effort as a control experiment:

In all seriousness, despite a lack of scientific rigor, I am willing to accept that the videos are broadly representative of the experiences many women have walking around. They demonstrate to me that the harassment is real, unsolicited, annoying, and occasionally terrifying. No one should have to put up with behavior like that and it is a terrible thing to be subjected to. We, as a society, need to figure out how to understand and manage this.

Other than the fact that all the harassers were men, one rather conspicuous thing jumped out at me while watching these videos: the men in the video seemed to be mentally and/or emotionally ill individuals. This in no way justifies their behavior and the harassment is clearly real. But seriously, what kind of person just starts randomly talking to another person about ANYTHING as they walk down the street, with no other context, demanding all of their attention? Someone who is mentally ill, practically by definition. Sure, talking to someone randomly on the street is occasionally appropriate. The annoying sales person can be given a legitimate excuse, even if frustrating. A panhandler is perhaps also in a special category (panhandling is not necessarily acceptable, but it is understood to a degree). Yes, the occasional “hello” or “have a good day” to a stranger might work when it is natural — which it usually isn’t while just walking down the street minding your own business. That they were mostly non-white men in Bliss’s video is likely a selection bias on the part of the editor. That they were men shows a clear testosterone connection.

In the videos, the perpetrators seem to be men who lack self control, who genuinely can’t manage their own impulses, physical and verbal, who don’t understand social conventions and basic etiquette. Self evidently, they are men who lack empathy or understanding of another person’s physical and emotional space. It is as if they have some kind of aggressive nervous tick they can’t control. The adult human mind is full of noise; there are impulses coming from many sectors of the psyche. However, most people, emotionally and mentally healthy adults, men and women alike of all walks of life, learn how to manage those internal impulses. Adults who can’t do that usually have some kind of brain damage, perhaps to the frontal lobe where impulse control is seated, or are not emotionally or mentally healthy in some other way.

A back-of-the-envelope calculation is worth doing. How many people does one expect to be in “interaction range” during a 10 hour excursion in New York City and what percentage is the observed 100 harassments of that number? This will help set the scale for the fraction of individuals harassing these women.

1) The population density of New York City: 26403 people per square mile ~ 0.01 people per square meter ~ 1 person per 100 square meters

2) 100 square meters might be regarded as a sensible “interactions zone” around a typical person walking around: +/- 5 meters in each direction

3) The typical walking speed of a person is around: 1.5 m/s

4) Imagine breaking New York City into a grid of 10 x 10 meter squares

5) The time to transverse 10 meters and move to one unique 10 square meter cell: about 6.67 seconds

6) There are 3600 seconds in 1 hour, so a 10 hour walk in NYC will sample about 5400 unique people on average in New York

7) If there were 100 harassment events/5400 persons during the walk in the video, this is about a 1.8% or 2% effect

That is, about 2% of the people Roberts interacted with during her excursion with Bliss harassed her to various degrees, violating her personal mental and emotional state. Again, this obviously isn’t scientific, but rather just a back-of-the-envelope. If I had to guess, I would say I underestimated the number of unique people per square meter one encounters on the street during the day in NYC. In other words, 2% is probably high.

If you asked me in advance “what fraction of people in New York City have mental problems involving a pathological lack of self control?” I would likely have guessed something like 10%. So, I could easily believe that the 2% number is looking at a subset of that that group, representing adult men whose mental illness, emotional illness, and excessive lack of self control is particularly aggressive and directed towards women. This 2% number then represents about 4% of the male population. This, I believe, is what these videos are measuring: a mental health problem specific to some men. It also explains the relative uniformity of the distribution across New York City, a point emphasized in William’s video.

The good news is, if it is a specific kind of mental health problem intrinsic to some population of men, and not some completely ill-defined problem, then perhaps this points to a strategy to help organizations like Hollaback end the awful street harassment many women experience.

Let me clarify that:
I’m in no way claiming that all harassment directed toward women across all social and cultural modes is due to mental illness alone; the causes of harassment are surely complex, perhaps involving trained dysfunctional socialized behaviors from early childhood, personality disorders, and other extensions of “healthy” mental states — but which are not a form of mental illness per se. I also hope that I have not given the impression that am I rationalizing away the effect or removing the element of personal responsibility from perpetrators. I’m merely proposing that one contribution to the problem — particularly in the context of aggressive street harassment of the sort shown in the video — may be a particular form of mental illness. I’m suggesting that scientifically exploring this contribution, by trained professionals, may be worthwhile.